The standard algorithm for solving such tasks involves, after finding the zeros of the function, the determination of the signs of the derivative on the intervals. Then the calculation of the values ​​at the found points of the maximum (or minimum) and on the border of the interval, depending on what question is in the condition.

I advise you to do things a little differently. Why? Wrote about it.

I propose to solve such tasks as follows:

1. Find the derivative.
2. Find the zeros of the derivative.
3. Determine which of them belong to the given interval.
4. We calculate the values ​​of the function on the boundaries of the interval and points of item 3.
5. We draw a conclusion (we answer the question posed).

In the course of solving the presented examples, the solution of quadratic equations is not considered in detail, you should be able to do this. They should also know.

Consider examples:

77422. Find the largest value of the function y=x 3 –3x+4 on the segment [–2;0].

Let's find the zeros of the derivative:

The point x = –1 belongs to the interval specified in the condition.

We calculate the function values ​​at points –2, –1 and 0:

The largest value of the function is 6.

Answer: 6

77425. Find the smallest value of the function y \u003d x 3 - 3x 2 + 2 on the segment.

Find the derivative of the given function:

Let's find the zeros of the derivative:

The point x = 2 belongs to the interval specified in the condition.

We calculate the function values ​​at points 1, 2 and 4:

The smallest value of the function is -2.

Answer: -2

77426. Find the largest value of the function y \u003d x 3 - 6x 2 on the segment [-3; 3].

Find the derivative of the given function:

Let's find the zeros of the derivative:

The point x = 0 belongs to the interval specified in the condition.

We calculate the function values ​​at points –3, 0 and 3:

The smallest value of the function is 0.

Answer: 0

77429. Find the smallest value of the function y \u003d x 3 - 2x 2 + x + 3 on the segment.

Find the derivative of the given function:

3x 2 - 4x + 1 = 0

We get the roots: x 1 \u003d 1 x 1 \u003d 1/3.

Only x = 1 belongs to the interval specified in the condition.

Find the function values ​​at points 1 and 4:

We found that the smallest value of the function is 3.

Answer: 3

77430. Find the largest value of the function y \u003d x 3 + 2x 2 + x + 3 on the segment [- 4; -one].

Find the derivative of the given function:

Find the zeros of the derivative, solve the quadratic equation:

3x 2 + 4x + 1 = 0

Let's get the roots:

The root х = –1 belongs to the interval specified in the condition.

Find the function values ​​at points –4, –1, –1/3 and 1:

We found that the largest value of the function is 3.

Answer: 3

77433. Find the smallest value of the function y \u003d x 3 - x 2 - 40x +3 on the segment.

Find the derivative of the given function:

Find the zeros of the derivative, solve the quadratic equation:

3x 2 - 2x - 40 = 0

Let's get the roots:

The root x = 4 belongs to the interval specified in the condition.

We find the values ​​of the function at points 0 and 4:

We found that the smallest value of the function is -109.

Answer: -109

Consider a method for determining the largest and smallest values ​​of functions without a derivative. This approach can be used if you have big problems with the definition of the derivative. The principle is simple - we substitute all integer values ​​from the interval into the function (the fact is that in all such prototypes the answer is an integer).

77437. Find the smallest value of the function y \u003d 7 + 12x - x 3 on the segment [-2; 2].

We substitute points from -2 to 2: View Solution

77434. Find the largest value of the function y \u003d x 3 + 2x 2 - 4x + 4 on the segment [-2; 0].

That's all. Good luck to you!

Sincerely, Alexander Krutitskikh.

P.S: I would be grateful if you tell about the site in social networks.

The process of finding the smallest and largest values ​​of a function on a segment is reminiscent of a fascinating flight around an object (a graph of a function) on a helicopter with firing from a long-range cannon at certain points and choosing from these points very special points for control shots. Points are selected in a certain way and according to certain rules. By what rules? We will talk about this further.

If the function y = f(x) continuous on the segment [ a, b] , then it reaches on this segment least and highest values . This can either happen in extremum points or at the ends of the segment. Therefore, to find least and the largest values ​​of the function , continuous on the segment [ a, b] , you need to calculate its values ​​in all critical points and at the ends of the segment, and then choose the smallest and largest of them.

Let, for example, it is required to determine the maximum value of the function f(x) on the segment [ a, b] . To do this, find all its critical points lying on [ a, b] .

critical point is called the point at which function defined, and her derivative is either zero or does not exist. Then you should calculate the values ​​of the function at critical points. And, finally, one should compare the values ​​of the function at critical points and at the ends of the segment ( f(a) and f(b) ). The largest of these numbers will be the largest value of the function on the interval [a, b] .

The problem of finding the smallest values ​​of the function .

We are looking for the smallest and largest values ​​​​of the function together

Example 1. Find the smallest and largest values ​​of a function on the segment [-1, 2] .

Solution. We find the derivative of this function. Equate the derivative to zero () and get two critical points: and . To find the smallest and largest values ​​of a function on a given segment, it is enough to calculate its values ​​at the ends of the segment and at the point , since the point does not belong to the segment [-1, 2] . These function values ​​are the following: , , . It follows that smallest function value(marked in red on the graph below), equal to -7, is reached at the right end of the segment - at the point , and greatest(also red on the graph), is equal to 9, - at the critical point .

If the function is continuous in a certain interval and this interval is not a segment (but is, for example, an interval; the difference between an interval and a segment: the boundary points of the interval are not included in the interval, but the boundary points of the segment are included in the segment), then among the values ​​of the function there may not be be the smallest and largest. So, for example, the function depicted in the figure below is continuous on ]-∞, +∞[ and does not have the largest value.

However, for any interval (closed, open, or infinite), the following property of continuous functions holds.

Example 4. Find the smallest and largest values ​​of a function on the segment [-1, 3] .

Solution. We find the derivative of this function as the derivative of the quotient:

.

We equate the derivative to zero, which gives us one critical point: . It belongs to the interval [-1, 3] . To find the smallest and largest values ​​of a function on a given segment, we find its values ​​at the ends of the segment and at the found critical point:

Let's compare these values. Conclusion: equal to -5/13, at the point and the greatest value equal to 1 at the point .

We continue to search for the smallest and largest values ​​​​of the function together

There are teachers who, on the topic of finding the smallest and largest values ​​of a function, do not give students examples more complicated than those just considered, that is, those in which the function is a polynomial or a fraction, the numerator and denominator of which are polynomials. But we will not limit ourselves to such examples, since among teachers there are lovers of making students think in full (table of derivatives). Therefore, the logarithm and the trigonometric function will be used.

Example 6. Find the smallest and largest values ​​of a function on the segment .

Solution. We find the derivative of this function as derivative of the product :

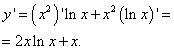
We equate the derivative to zero, which gives one critical point: . It belongs to the segment. To find the smallest and largest values ​​of a function on a given segment, we find its values ​​at the ends of the segment and at the found critical point:

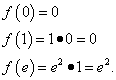
The result of all actions: the function reaches its minimum value, equal to 0, at a point and at a point and the greatest value equal to e² , at the point .

Example 7. Find the smallest and largest values ​​of a function on the segment .

Solution. We find the derivative of this function:

Equate the derivative to zero:

The only critical point belongs to the segment . To find the smallest and largest values ​​of a function on a given segment, we find its values ​​at the ends of the segment and at the found critical point:

Conclusion: the function reaches its minimum value, equal to , at the point and the greatest value, equal to , at the point .

In applied extremal problems, finding the smallest (largest) function values, as a rule, is reduced to finding the minimum (maximum). But it is not the minima or maxima themselves that are of greater practical interest, but the values ​​of the argument at which they are achieved. When solving applied problems, an additional difficulty arises - the compilation of functions that describe the phenomenon or process under consideration.

Example 8 A tank with a capacity of 4, having the shape of a parallelepiped with a square base and open at the top, must be tinned. What should be the dimensions of the tank in order to cover it with the least amount of material?

Solution. Let x- base side h- tank height, S- its surface area without cover, V- its volume. The surface area of ​​the tank is expressed by the formula , i.e. is a function of two variables. To express S as a function of one variable, we use the fact that , whence . Substituting the found expression h into the formula for S:

Let us examine this function for an extremum. It is defined and differentiable everywhere in ]0, +∞[ , and

.

We equate the derivative to zero () and find the critical point. In addition, at , the derivative does not exist, but this value is not included in the domain of definition and therefore cannot be an extremum point. So, - the only critical point. Let's check it for the presence of an extremum using the second sufficient sign. Let's find the second derivative. When the second derivative is greater than zero (). This means that when the function reaches a minimum . Because this minimum - the only extremum of this function, it is its smallest value. So, the side of the base of the tank should be equal to 2 m, and its height.

Example 9 From paragraph A, located on the railway line, to the point WITH, at a distance from it l, goods must be transported. The cost of transporting a weight unit per unit distance by rail is equal to , and by highway it is equal to . To what point M railroad line should be held highway to transport cargo from A v WITH was the most economical AB railroad is assumed to be straight)?

The largest and smallest value of the function

The largest value of a function is called the largest, the smallest value is the smallest of all its values.

A function may have only one largest and only one smallest value, or may not have any at all. Finding the largest and smallest values ​​of continuous functions is based on the following properties of these functions:

1) If in some interval (finite or infinite) the function y=f(x) is continuous and has only one extremum, and if this is the maximum (minimum), then it will be the largest (smallest) value of the function in this interval.

2) If the function f(x) is continuous on some segment , then it necessarily has the largest and smallest values ​​on this segment. These values ​​are reached either at the extremum points lying inside the segment, or at the boundaries of this segment.

To find the largest and smallest values ​​on the segment, it is recommended to use the following scheme:

1. Find the derivative.

2. Find the critical points of the function where =0 or does not exist.

3. Find the values ​​of the function at critical points and at the ends of the segment and choose from them the largest f max and the smallest f min.

When solving applied problems, in particular optimization problems, the problems of finding the largest and smallest values ​​(global maximum and global minimum) of a function on the interval X are important. To solve such problems, one should, based on the condition, choose an independent variable and express the value under study through this variable. Then find the desired maximum or minimum value of the resulting function. In this case, the interval of change of the independent variable, which can be finite or infinite, is also determined from the condition of the problem.

Example. The tank, which has the shape of a rectangular parallelepiped with a square bottom, open at the top, must be tinned inside with tin. What should be the dimensions of the tank with a capacity of 108 liters. water so that the cost of its tinning is the least?

Solution. The cost of coating the tank with tin will be the lowest if, for a given capacity, its surface is minimal. Denote by a dm - the side of the base, b dm - the height of the tank. Then the area S of its surface is equal to

AND

The resulting relation establishes the relationship between the surface area of ​​the tank S (function) and the side of the base a (argument). We investigate the function S for an extremum. Find the first derivative, equate it to zero and solve the resulting equation:

Hence a = 6. (a) > 0 for a > 6, (a)< 0 при а < 6. Следовательно, при а = 6 функция S имеет минимум. Если а = 6, то b = 3. Таким образом, затраты на лужение резервуара емкостью 108 литров будут наименьшими, если он имеет размеры 6дм х 6дм х 3дм.

Example. Find the largest and smallest values ​​of a function in between.

Solution: The specified function is continuous on the entire number axis. Function derivative

Derivative at and at . Let's calculate the values ​​of the function at these points:

.

The function values ​​at the ends of the given interval are equal to . Therefore, the largest value of the function is at , the smallest value of the function is at .

Questions for self-examination

1. Formulate L'Hopital's rule for disclosure of uncertainties of the form . List the different types of uncertainties for which L'Hospital's rule can be used.

2. Formulate signs of increasing and decreasing function.

3. Define the maximum and minimum of a function.

4. Formulate the necessary condition for the existence of an extremum.

5. What values ​​of the argument (what points) are called critical? How to find these points?

6. What are sufficient signs of the existence of an extremum of a function? Outline a scheme for studying a function for an extremum using the first derivative.

7. Outline the scheme for studying the function for an extremum using the second derivative.

8. Define convexity, concavity of a curve.

9. What is the inflection point of a function graph? Specify how to find these points.

10. Formulate the necessary and sufficient signs of convexity and concavity of the curve on a given segment.

11. Define the asymptote of the curve. How to find the vertical, horizontal and oblique asymptotes of a function graph?

12. Outline the general scheme for researching a function and constructing its graph.

13. Formulate a rule for finding the largest and smallest values ​​of a function on a given interval.

What is an extremum of a function and what is the necessary condition for an extremum?

The extremum of a function is the maximum and minimum of the function.

The necessary condition for the maximum and minimum (extremum) of the function is as follows: if the function f(x) has an extremum at the point x = a, then at this point the derivative is either zero, or infinite, or does not exist.

This condition is necessary, but not sufficient. The derivative at the point x = a can vanish, go to infinity, or not exist without the function having an extremum at this point.

What is the sufficient condition for the extremum of the function (maximum or minimum)?

First condition:

If, in sufficient proximity to the point x = a, the derivative f?(x) is positive to the left of a and negative to the right of a, then at the point x = a itself, the function f(x) has maximum

If, in sufficient proximity to the point x = a, the derivative f?(x) is negative to the left of a and positive to the right of a, then at the point x = a itself, the function f(x) has minimum provided that the function f(x) is continuous here.

Instead, you can use the second sufficient condition for the extremum of the function:

Let at the point x = and the first derivative f? (x) vanishes; if the second derivative f??(а) is negative, then the function f(x) has a maximum at the point x = a, if it is positive, then a minimum.

What is the critical point of a function and how to find it?

This is the value of the function argument at which the function has an extremum (i.e. maximum or minimum). To find it, you need find the derivative function f?(x) and, equating it to zero, solve the equation f?(x) = 0. The roots of this equation, as well as those points at which the derivative of this function does not exist, are critical points, i.e., the values ​​of the argument at which there may be an extremum. They can be easily identified by looking at derivative graph: we are interested in those values ​​of the argument at which the graph of the function intersects the abscissa axis (Ox axis) and those at which the graph suffers breaks.

For example, let's find extremum of the parabola.

Function y(x) = 3x2 + 2x - 50.

Function derivative: y?(x) = 6x + 2

We solve the equation: y?(x) = 0

6x + 2 = 0, 6x = -2, x = -2/6 = -1/3

In this case, the critical point is x0=-1/3. It is for this value of the argument that the function has extremum. To get it find, we substitute the found number in the expression for the function instead of "x":

y0 = 3*(-1/3)2 + 2*(-1/3) - 50 = 3*1/9 - 2/3 - 50 = 1/3 - 2/3 - 50 = -1/3 - 50 = -50.333.

How to determine the maximum and minimum of a function, i.e. its largest and smallest values?

If the sign of the derivative changes from “plus” to “minus” when passing through the critical point x0, then x0 is maximum point; if the sign of the derivative changes from minus to plus, then x0 is minimum point; if the sign does not change, then at the point x0 there is neither a maximum nor a minimum.

For the considered example:

We take an arbitrary value of the argument to the left of the critical point: x = -1

When x = -1, the value of the derivative will be y? (-1) = 6 * (-1) + 2 = -6 + 2 = -4 (i.e., the minus sign).

Now we take an arbitrary value of the argument to the right of the critical point: x = 1

For x = 1, the value of the derivative will be y(1) = 6 * 1 + 2 = 6 + 2 = 8 (i.e., the plus sign).

As you can see, when passing through the critical point, the derivative changed sign from minus to plus. This means that at the critical value of x0 we have a minimum point.

The largest and smallest value of the function on the interval(on the segment) are found by the same procedure, only taking into account the fact that, perhaps, not all critical points will lie within the specified interval. Those critical points that are outside the interval must be excluded from consideration. If there is only one critical point inside the interval, it will either have a maximum or a minimum. In this case, to determine the largest and smallest values ​​of the function, we also take into account the values ​​of the function at the ends of the interval.

For example, let's find the largest and smallest values ​​of the function

y (x) \u003d 3 sin (x) - 0.5x

at intervals:

So the derivative of the function is

y?(x) = 3cos(x) - 0.5

We solve the equation 3cos(x) - 0.5 = 0

cos(x) = 0.5/3 = 0.16667

x \u003d ± arccos (0.16667) + 2πk.

We find critical points on the interval [-9; 9]:

x \u003d arccos (0.16667) - 2π * 2 \u003d -11.163 (not included in the interval)

x \u003d -arccos (0.16667) - 2π * 1 \u003d -7.687

x \u003d arccos (0.16667) - 2π * 1 \u003d -4.88

x \u003d -arccos (0.16667) + 2π * 0 \u003d -1.403

x \u003d arccos (0.16667) + 2π * 0 \u003d 1.403

x \u003d -arccos (0.16667) + 2π * 1 \u003d 4.88

x \u003d arccos (0.16667) + 2π * 1 \u003d 7.687

x \u003d -arccos (0.16667) + 2π * 2 \u003d 11.163 (not included in the interval)

We find the values ​​of the function at critical values ​​of the argument:

y(-7.687) = 3cos(-7.687) - 0.5 = 0.885

y(-4.88) = 3cos(-4.88) - 0.5 = 5.398

y(-1.403) = 3cos(-1.403) - 0.5 = -2.256

y(1.403) = 3cos(1.403) - 0.5 = 2.256

y(4.88) = 3cos(4.88) - 0.5 = -5.398

y(7.687) = 3cos(7.687) - 0.5 = -0.885

It can be seen that on the interval [-9; 9] the function has the greatest value at x = -4.88:

x = -4.88, y = 5.398,

and the smallest - at x = 4.88:

x = 4.88, y = -5.398.

On the interval [-6; -3] we have only one critical point: x = -4.88. The value of the function at x = -4.88 is y = 5.398.

We find the value of the function at the ends of the interval:

y(-6) = 3cos(-6) - 0.5 = 3.838

y(-3) = 3cos(-3) - 0.5 = 1.077

On the interval [-6; -3] we have the largest value of the function

y = 5.398 at x = -4.88

the smallest value is

y = 1.077 at x = -3

How to find the inflection points of a function graph and determine the sides of convexity and concavity?

To find all the inflection points of the line y \u003d f (x), you need to find the second derivative, equate it to zero (solve the equation) and test all those values ​​of x for which the second derivative is zero, infinite or does not exist. If, when passing through one of these values, the second derivative changes sign, then the graph of the function has an inflection at this point. If it does not change, then there is no inflection.

The roots of the equation f ? (x) = 0, as well as possible points of discontinuity of the function and the second derivative, divide the domain of the function into a number of intervals. The convexity at each of their intervals is determined by the sign of the second derivative. If the second derivative at a point on the interval under study is positive, then the line y = f(x) is concave upwards here, and if it is negative, then downwards.

How to find extrema of a function of two variables?

To find the extrema of the function f(x, y), differentiable in the area of ​​its assignment, you need:

1) find the critical points, and for this, solve the system of equations

fx? (x,y) = 0, fy? (x,y) = 0

2) for each critical point P0(a;b), investigate whether the sign of the difference remains unchanged

for all points (x; y) sufficiently close to P0. If the difference retains a positive sign, then at the point P0 we have a minimum, if negative, then a maximum. If the difference does not retain its sign, then there is no extremum at the point Р0.

Similarly, the extrema of the function are determined for a larger number of arguments.

Let's see how to explore a function using a graph. It turns out that looking at the graph, you can find out everything that interests us, namely:

• function scope
• function range
• function zeros
• periods of increase and decrease
• high and low points
• the largest and smallest value of the function on the segment.

Let's clarify the terminology:

Abscissa is the horizontal coordinate of the point.
Ordinate- vertical coordinate.
abscissa- the horizontal axis, most often called the axis.
Y-axis- vertical axis, or axis.

Argument is an independent variable on which the values ​​of the function depend. Most often indicated.
In other words, we ourselves choose , substitute in the function formula and get .

Domain functions - the set of those (and only those) values ​​of the argument for which the function exists.
Denoted: or .

In our figure, the domain of the function is a segment. It is on this segment that the graph of the function is drawn. Only here this function exists.

Function range is the set of values ​​that the variable takes. In our figure, this is a segment - from the lowest to the highest value.

Function zeros- points where the value of the function is equal to zero, i.e. . In our figure, these are the points and .

Function values ​​are positive where . In our figure, these are the intervals and .
Function values ​​are negative where . We have this interval (or interval) from to.

The most important concepts - increasing and decreasing function on some set. As a set, you can take a segment, an interval, a union of intervals, or the entire number line.

Function increases

In other words, the more , the more , that is, the graph goes to the right and up.

Function decreases on the set if for any and belonging to the set the inequality implies the inequality .

For a decreasing function, a larger value corresponds to a smaller value. The graph goes right and down.

In our figure, the function increases on the interval and decreases on the intervals and .

Let's define what is maximum and minimum points of the function.

Maximum point- this is an internal point of the domain of definition, such that the value of the function in it is greater than in all points sufficiently close to it.
In other words, the maximum point is such a point, the value of the function at which more than in neighboring ones. This is a local "hill" on the chart.

In our figure - the maximum point.

Low point- an internal point of the domain of definition, such that the value of the function in it is less than in all points sufficiently close to it.
That is, the minimum point is such that the value of the function in it is less than in neighboring ones. On the graph, this is a local “hole”.

In our figure - the minimum point.

The point is the boundary. It is not an interior point of the domain of definition and therefore does not fit the definition of a maximum point. After all, she has no neighbors on the left. In the same way, there can be no minimum point on our chart.

The maximum and minimum points are collectively called extremum points of the function. In our case, this is and .

But what if you need to find, for example, function minimum on the cut? In this case, the answer is: because function minimum is its value at the minimum point.

Similarly, the maximum of our function is . It is reached at the point .

We can say that the extrema of the function are equal to and .

Sometimes in tasks you need to find the largest and smallest values ​​of the function on a given segment. They do not necessarily coincide with extremes.

In our case smallest function value on the interval is equal to and coincides with the minimum of the function. But its largest value on this segment is equal to . It is reached at the left end of the segment.

In any case, the largest and smallest values ​​of a continuous function on a segment are achieved either at the extremum points or at the ends of the segment.